Physics research in twelve one-minute pieces
Enjoy these twelve pieces of street physics just like you enjoy street music: come along, listen to a piece, enjoy it – or not – and continue on your way. The pieces were inspired by the summary of physics in nine lines. Together, the twelve pieces show how to present and unify physics in just one line.
1
2There is a maximum energy speed in nature
3There is a smallest observable change in nature
4There is a maximum force in nature
5Strands explain quantum theory
6Strands explain general relativity and
quantum gravity
7Strands explain particles
8Strands explain gauge interactions
9Strands explain fundamental constants
10Strands yield cosmology
11Strands explain all motion
12The garden at the end of the street
Slides
Bibliography
Strings vs. Strands
1 Nature is maximally efficient
Teaser.
Everything around us happens in a way that minimizes change.Exploration.
It took physicists many years to sort out the definition of "change". It was finalized around 1750.A system with a lot of kinetic energy moves a lot. The more time passes, the more the system changes. Thus, change increases both with kinetic energy and with time.
A system that stores energy reduces motion. Potential energy reduces change, and the more the longer it is stored. Thus, change decreases both with potential energy and with time.
Therefore, physical "change" is kinetic energy minus potential energy, multiplied by the elapsed time. But physicists do not say "change" – they say "action". Think about an action movie. A large action value is for movies with a "lot of action". When many cars or planes move, explode, or burn, then the action value – the integral over time of kinetic minus potential energy – is large. (For general relativity, this expression of the action is generalized.)
All equations of motion follow from the requirement that action, defined accordingly, is minimized. No counter-example is known.
Summary.
Motion minimizes change. This is officially called the principle of least action. Everything that happens follows this rule, from the growth of trees to the motion of lightning. In simple English: nature is efficient; it dislikes squandering.Possible falsification.
Find an exception to least action.More to ponder.
Action W is the most fundamental quantity in physics. The 10-volume Landau-Lifshitz textbook on physics starts with action on the very first page. Efficiency applies to every process in nature: action minimization is valid in classical physics, in quantum theory and in general relativity. It is valid for motion of stones, heat, light, particles, fields and empty space.In short,
δW = 0 describes motion and agrees with observations.2 There is a maximum energy speed in nature
Teaser.
Nature dislikes being in a hurry.Exploration.
It took physicists many years to discover that there is a speed limit for energy. It was clarified in the early 20th century.Throwing a stone while running yields a greater speed than throwing it standing still. However, in a vacuum, light from a moving lamp is not faster than light from a lamp at rest.
Even extremely light "stones", single electrons, cannot be accelerated faster than light, even using the largest amounts of energy. This also applies to protons, neutrinos, rocks, rockets, X-rays or gravitational waves.
Despite high rewards, nobody has found a way to move energy faster than light in a vacuum.
Summary.
The speed of light in a vacuum is the limit for the speed of any kind of energy. This is the principle on which special relativity is based. In simple language: nature refuses to hurry.Possible falsification.
Find a larger local energy speed.More to ponder.
The speed limit states that the locally measured energy speed value, relative to the observer, never exceeds c. Also, the observer performing the measurement must be physical and, e.g., cannot be superluminal. "Geometric" speeds can be higher than c, such as the speed of shadows, images or horizons.The speed limit c is so fundamental that it is used to define the meter in terms of the second. The speed limit also means that time is different for different observers that move against each other. The principle of maximum local energy speed implies the full theory of special relativity.
In water, particles can move faster than light. What happens?
In short,
v ≤ c implies special relativity and agrees with observations.3 There is a smallest observable change in nature
Teaser.
Nature dislikes sloth. Something happens all the time.Exploration.
In the 1890s, Planck discovered photons, the particles of light. He also found that it is not possible to measure smaller changes than ħ, a constant of nature that he called the elementary quantum of action. (Actually, he found that the smallest change was h=2πħ, but nowadays the two quantities are often used interchangeably.)The term "quantum" was made popular by Galileo, who explained that matter is made of "piccolissimi quanti", tiny quanta that are not divisible. Planck took over the term. He was a quiet man but was conscious that he made, by chance, one of the greatest discoveries ever.
Attempt of a counter-example. Determining the action of a free particle requires measuring its energy at two different points in time. Even though, classically, action W is given by energy E times delta t, in nature and in quantum theory the action value W remains finite when delta t gets small, because of the uncertainty relation: the energy (difference) increases when delta t decreases. (Measuring energy requires time, and that time must be shorter than delta t.) For small delta t, ħ is at work: the uncertainty relation prevents that the measured action goes to zero when delta t goes to zero.
Further attempt of a counter-example. Detecting a spin 1/2 flip requires an action ℏ. There is no way to detect a spin flip with a smaller amount of action.
Another attempt. Every photon detection requires an action ℏ. In nature, there is just no way to detect one-half or one-hundredth of a photon. Photons are elementary: they cannot be split.
The famous discussion between Einstein and Bohr can be seen as a repeated attempt by Einstein to measure actions below ħ. But nature does not allow this. Einstein, who in the beginning was sure that this was possible, was convinced. There is a lower limit to action.
Summary.
In 1899, quantum theory was born with Planck's discovery that action is quantized in multiples of the minimum measurable value ħ. The principle of quantized action was confirmed by all subsequent experiments.Possible falsification.
Measure a smaller action value than the lower limit given by the quantum of action.More to ponder.
If ħ were not the smallest action value, atoms would not exist.For physicists. Action is a scalar, a Lorentz invariant, and a quantum observable. The operator, found in textbooks, is self-adjoint and linear. There is also a canonically conjugated observable: angle.
Probabilities arise whenever one approaches the limit. The limit ħ alone is sufficient to deduce wave functions and quantum mechanics.
In short,
W ≥ ħ implies quantum theory and agrees with observations.4 There is a maximum force in nature
Teaser.
Nature dislikes excessive force. There is a limit to force.In nature, c is the maximum local energy speed. Similarly, c4/4G is the maximum local force, or maximum local momentum flow.
Exploration.
The force limit c4/4G implies that the locally measured force value, relative to the observer, never exceeds c4/4G. The observer performing the measurement must be physical and, e.g., cannot be a black hole, or infinitesimally dense, or infinitesimally small. These statements can also be repeated for the maximum power or luminosity c5/4G. This was clarified in the 1990s and 2000s.Attempts to find counterexamples must be physical. An observer cannot add forces of distant mass configurations and claim that their sum exceeds the local limit c4/4G. Such examples are easily found. It is thus wrong to claim that maximum force is valid for any physical surface.
The value c4/4G is the largest possible gravitational force between two black holes.
The maximum force value implies inverse square gravity.
So far, no counterexamples to the maximum luminosity c5/4G have been published. Even the most recent observations of black hole mergers fail to exceed the luminosity limit. The highest instantaneous luminosity observed so far is about 0.5% of the maximum value.
Summary.
No counter-examples or counter-arguments to maximum force or to maximum power have yet been observed or constructed.The existence of a local maximum force is sufficient to deduce general relativity in its entirety, including the field equations. This is the principle of maximum force.
Possible falsification.
Find a larger local force or luminosity.More to ponder.
Can you find a way to distinguish experimentally whether the limit is c4/G or c4/4G?Maximum force also applies to electromagnetism and nuclear interactions.
A maximum force also implies that space is curved.
A maximum force implies that perfectly straight motion does not exist.
In short,
F ≤ c4/4G implies general relativity and agrees with observations.5 Strands explain quantum theory
Teaser.
Only tethered structures can be matter particles.Dirac's equation follows from invisible fluctuating strands.
Exploration.
Dirac showed, around 1929, that matter particles, i.e., spin 1/2 particles, behave like tethered objects: they come back to the original state only after rotations by 4π, not after rotation by 2π. After a 2π rotation, the tethers remain tangled.Such tethered particles can rotate continuously.
In nature, a rotation by 2π leads to a minus sign in front of the wave function. A rotation by 4π leads to a plus sign.
Tethers with Planck radius realize the minimum length as well as the extension of wave functions and of space.
Simply said, nature does not allow us to observe tethers, but does allow us to observe their crossing switches. And the crossing switches of invisible (i.e., Planck-radius) tethers define ħ and c.
In 1980, Battey-Pratt and Racey showed that a Lorentz transformation of this connection yields Dirac's equation. In 1987, Kauffman suggested a relation between crossing switches and ħ.
Tethered particles behave as fermions: the system goes back to its original state only after a double particle exchange. Tethers confirm the spin-statistics theorem.
The geometric variables describing a strand crossing – position, density, orientation, phase – are the same variables that describe a wave function.
Dirac's trick also implies that the wave function is the average crossing density of invisible fluctuating tethers. We call them strands.
Summary.
Dirac's trick implies Dirac's equation. Strands imply wave functions.Possible falsification.
Find a deviation from the Dirac equation, e.g., at high energy or for high gravitational fields.More to ponder.
Strands do not provide hidden variables.Strand entanglement explains quantum entanglement.
Particle rotation, or phase rotation, is the reason for the appearance of complex numbers in quantum theory.
In short,
wave functions are blurred strand crossings. Tangles are skeletons of wave functions. This agrees with observations. More in6 Strands explain general relativity and quantum gravity
Teaser.
Nature dislikes boredom - and flat space in particular. Einstein's field equations follow from invisible fluctuating strands of Planck radius.Exploration.
Crossing switches of strands realize the limits c, c4/4G and the Boltzmann constant k.Horizons are tight weaves of strands. Flat vacuum is made of untangled strands. Curvature is an inhomogeneous strand distribution.
There is a smallest length in nature. Strands realize the minimum length and thus explain black hole entropy, temperature and energy. These relations allow deducing Einstein's field equations. This was discovered around 2010 using a result by Jacobson from 1995.
The Hilbert Lagrangian of general relativity thus follows from strands.
There is no gravitational physics beyond general relativity at sub-galactic scales.
Space has three dimensions because strand tangles do not exist in other dimensions.
Gravitons are made of two strands; they have spin 2 and vanishing mass.
On average, masses are surrounded by twisted tethers; the twists are due to Dirac's trick. The twisted tethers form clouds around every mass and behave as expected from virtual gravitons: clouds of twists lead to attraction between masses. For small curvature and speeds, and when no horizon is nearby, these graviton clouds imply universal 1/r2 gravitation.
Single gravitons are not detectable.
New quantum gravity effects are not observable.
Again, because of the smallest length, nothing in nature is continuous or discrete.
Summary.
Dirac's trick implies Einstein's field equations. Strands make up space and gravity.Possible falsification.
Find a deviation from Einstein's field equations at sub-galactic scales. Measure any (new) effect of quantum gravity. Find non-commutative space. Find other dimensions.More to ponder.
Rotating black holes perform Dirac's trick with a huge number of tethers.A mass is never smaller than its own black hole radius. Black holes have a finite moment of inertia.
There are no singularities. There are no physical points in space. Space is only continuous after averaging out strands.
Strand topology and strand crossings lead to entropy and thus to gravitational dynamics.
In short,
gravitons are moving twisted strand pairs. This agrees with observations. See C. Schiller, Testing a conjecture on the origin of space, gravity and mass, Indian Journal of Physics 96 (2022) 3047–3064.7 Strands explain particles
Teaser.
Particles are not points, but tethered to the rest of the world.Moving particles are rotating rational tangles.
Exploration.
Rational tangles imply three generations of elementary fermions, each with two particles.Localized fermion rational tangles of two strands are quarks.
Localized fermion rational tangles of three strands are leptons.
(Localized 3-braids are Higgs bosons; two other localized three-tangles are W bosons and Z bosons.)
Each fermion is represented by an infinite family of rational tangles: a basic tangle and further family members that arise by the addition of braids.
Tangle topology determines all quantum numbers: spin, parities, charges and flavours.
There is no particle beyond the standard model. This was clarified in the years around 2010.
Summary.
Particles are spinning defects in space.Possible falsification.
Find any new elementary particle. Find a different particle model that agrees with experiment. Find a new quantum number. Find new physics.More to ponder.
Only rational tangles reproduce how particles change quantum numbers when they interact. Other topological structures – such as knots, ribbons, graphs or even just other types of tangles – do not match observations.Particles are not point-like, but at least of Planck size.
So far, no other model explains the particle spectrum.
In short,
elementary particles arise from the classification of tangles. Elementary particles and space are complementary. This agrees with observations. See8 Strands explain gauge interactions
Teaser.
Interactions of particles are due to strand deformations of tangles.Exploration.
In mathematics, deformations are described by gauge groups.All tangle deformations can be mathematically classified into three types, called the three Reidemeister moves. This was discovered in 1926.
The three Reidemeister moves determine U(1), broken SU(2), and SU(3). This was found about 80 years later.
Tangle deformation implies a change of phase – this effect is required from any interaction.
The three Reidemeister moves also determine the strand structure of the gauge bosons – photons, W, Z, and gluons.
There is no interaction and no symmetry beyond the standard model.
Summary.
The three known gauge groups derive from the three Reidemeister moves.Possible falsification.
Find another interaction, another gauge group, another fundamental symmetry or another gauge boson.More to ponder.
No other symmetry, gauge boson or interaction is predicted to exist. No supersymmetry, no unified gauge group, no Clifford algebra and no other internal space can describe nature.So far, no other model explains the interaction spectrum.
In short,
bending strands imply the observed gauge interactions. This agrees with observations. See9 Strands explain fundamental constants
Teaser.
Tangle topology and shape fluctuations determine interaction strengths.Exploration.
Deformation transfers explain Feynman diagrams. The transfer of Reidemeister moves between bosons and fermions explains the coupling constants.Dirac's trick explains masses – including neutrino masses.
Exchanges of tether positions explain mixing angles.
The Lagrangian of the standard model of elementary particle physics follows from strands. This was discovered around 2010.
Summary.
Tangle shapes determine the fundamental constants.Possible falsification.
Find a difference between calculated and measured values of the fundamental constants. Find a different explanation for the fundamental constants.More to ponder.
Average tangle shape is determined by tight tangle shape. But no non-trivial tight tangle shape is yet known analytically.There is no multiverse.
So far, no other model explains interaction strengths.
In short,
the hardest problem of 3d geometry - calculating the shape of tangles - is related to the hardest problem in physics: the fundamental constants. This agrees with observations. On the fine structure constant, see C. Schiller, Testing a conjecture on quantum electrodynamics, Journal of Geometry and Physics 178 (2022) 104551.10 Strands yield cosmology
Teaser.
The universe plays cat's cradle.Exploration.
The universe consists of a closed strand that continuously increases in complexity. The increase defines time.The universe is surrounded by a horizon. Strands solve the flatness, homogeneity and isotropy problems; also, there is nothing behind the cosmological horizon.
Empty space expands, because with time, more strand segments enter its inside from the horizon.
Empty space contains such a low energy density that dark energy vanishes. Despite Nobel prizes, the expansion of the universe does not accelerate, as David Wiltshire shows.
Because strands do not allow other defects than the known elementary particles, there is no elementary dark matter particle.
Strands imply the lack of inflation, because there is no mechanism for such a process.
Summary.
The sky is dark at night. There is dark energy – but its time dependence is not clear yet. There is no elementary dark matter. Despite appearances, the inverse square law is not modified at galactic and larger scales – as also shown by David Wiltshire.Possible falsification.
Find elementary dark matter. Find anything beyond the cosmological horizon. Find a modification to inverse square gravity.More to ponder.
Could the universe be made of several strands?Similar ideas on the structure of nature were proposed around 1990 by Lou Kauffman.
In short,
the universe consists of one closed strand. This agrees with observations.11 Strands explain all motion
Teaser.
No observed example of motion is unexplained.Exploration.
Strand tangles explain the three dimensions of space, the three gauge interactions and the three particle generations.Strand tangles explain the curvature of space and wave functions.
Strands explain all Lagrangians used in physics: that of general relativity and that of the standard model of particle physics. Without modification.
Local motion follows the principle of least crossing switch number, because crossing switches couple to photons. This explains, for the first time ever, the principle of least action.
Strands explain all mathematical concepts used in physics.
Strands explain that nature follows rules.
Summary.
No observation about motion in fundamental physics seems to be unexplained by strands. Strands explain and describe nature, physics and all the natural sciences.Possible falsification.
Find an example of motion that differs from the strand tangle model. Find additional dimensions.More to ponder.
Topology leads to shapes, deformations and to dynamics. Or: dynamics results from algebra.Is the strand explanation of nature satisfying? Does it fit on a T-shirt with sufficient elegance? Does it lead to say, like Wheeler requested, “Oh, how could it have been otherwise! How could we all have been so blind so long!”?
Strands do not need to have a constant radius.
Alternatively, instead of strands, the space between strands can be taken as the fundamental evolving entity, as done by Asselmeyer-Maluga.
In short,
motion locally minimizes crossing switches. This explains the existence of the observed Lagrangians and of three observed dimensions. On a cosmological scale, the number of crossing switches increases continuously.12 The garden at the end of the street
Teaser.
The tangle model implies all of nature and physics - and explains all colours.Exploration.
Nature likes motion. Everything that happens in nature is a kind of motion. Also all colours.To describe motion with precision, use the speed limit v≤c, the action limit W≥ħ and the force limit F≤c⁴/4G: this yields quantum theory, general relativity and cosmology. Realize the limits with crossing switches of strands with Planck radius, form rational tangles, and deform them with Reidemeister moves: this yields the particles, the interactions, the constants and the Lagrangian of the standard model.
Every equation of physics follows and emerges from strands.
Every type of motion, every measurement, and every observation follows from strands - and from minimizing crossing switches.
Strands and the Dirac trick at Planck scales together describe nature with full accuracy and full precision. Strands explain the three dimensions.
The 9 lines summarizing textbook physics follow from strands.
More precisely: tangled strands so far are the only approach in the literature that explains the first 8 lines summarizing physics, i.e., the only approach that explains the gauge groups and the particle spectrum, without adding anything. In addition, strands so far are the only approach that allows calculating the fundamental constants, i.e., the only approach that explains the 9th line. Strands predict that these calculations will agree with measurements. Strands are the only approach that already produced estimates for several fundamental constants which are not in contrast with experiments. This includes the fine structure constant 1/137.036(1) that determines all colours in nature, and various particle masses.
Conclusion.
Tangled strands describe accurately everything that happens. Tangles provide the tiniest theory of nature. Tangles explain all colours.Possible falsification.
Find a single difference between observations and the tangle model. Find physics beyond the standard model. Find a simpler model than the tangle model. Find a different model. Find an unexplained colour.More to ponder.
Strands imply: everything is connected to everything else. When you move, you carry many tethers along with you.Strands imply: every thing is made of everything.
Strands imply: the natural numbers are approximations.
One-line summary:
Nature is a tangle of a long, invisible, fluctuating strand whose crossing switches define the Planck units, all colours and all other observations. This yields full agreement with observations.Slides introducing strands
Pedagogical introduction for physicists
![]() The text derives wave functions,
particles and interactions from strands.
The text derives wave functions,
particles and interactions from strands.
Uniqueness of strands - for physicists
Bibliographic references about strands
Strands provide the only unified description of motion, as shown in
this text. ![]()
E.P. Battey-Pratt and T.J. Racey, Geometric model for fundamental particles, International Journal of Theoretical Physics 19 (1980) 437-475. They were the first researchers who thought about tethers/strands and derived Dirac's equation from them.
C. Schiller, A conjecture on deducing general relativity and the standard model with its fundamental constants from rational tangles of strands, Physics of Particles and Nuclei 50 (2019) 259-299. Details here.
C. Schiller, Testing a conjecture on the origin of the standard model, European Physical Journal Plus 136 (2021) 79. Details here.
Jason Hise's animations are shown and strands are mentioned in this PBS television program, from 8:48 onwards (2021).
C. Schiller, Testing a conjecture on the origin of space, gravity and mass, Indian Journal of Physics (2021). Download here.
A complete bibliography is found here.
Bibliographic references about the quantum of action
See the dedicated page on the quantum of action.
Bibliographic references about maximum force
See the dedicated page on maximum force.
Strings vs. strands
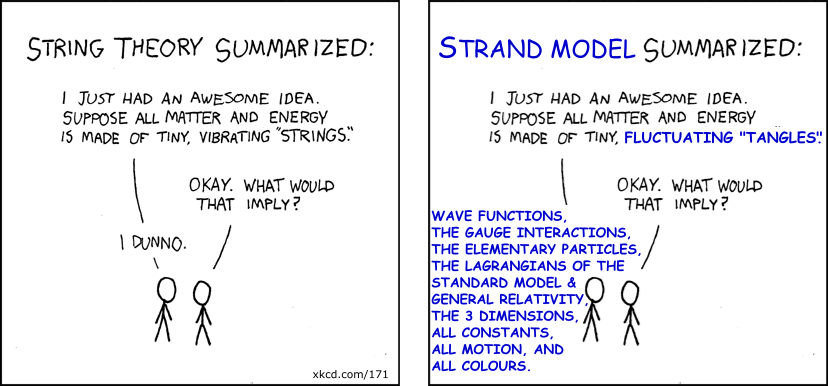
A comparison between the two approaches with proposals to
reduce the difference is found in the appendix of the preprint
![]() Testing a model for emergent spinor wave functions
explaining elementary particles and gauge interactions.
Testing a model for emergent spinor wave functions
explaining elementary particles and gauge interactions.